Picture
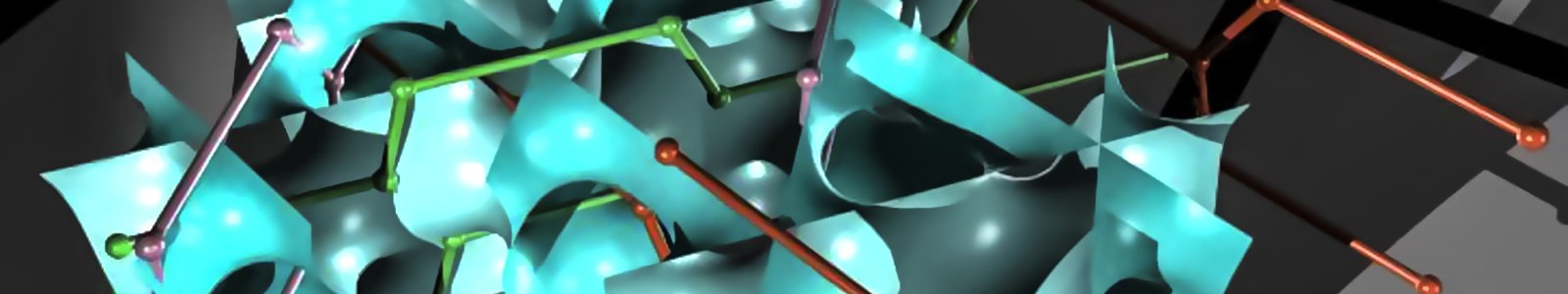
Schwarz hexagonal surface with embedded graphs
Image by Stuart Ramsden, Australian National University.
The picture shows a piece of the Schwarz hexagonal surface. This surface extends indefinitely in all three spacial directions. It is a minimal surface. This means that it locally has the shape of a soap film spanning a suitably bent frame. It also has a hyperbolic structure. Thus there is a covering map from the hyperbolic plane onto the hexagonal surface.
One may construct a forest in the hyperbolic plane. The covering map sends it to a graph in the hexagonal surface. This graph has been rendered in a stylised fashion. In reality some edges are bent, as they follow the surface. Different colors have been asigned to different trees in the forest. Different trees in the hyperbolic plane are not entangled, but their images in the hexagonal surface are entangled.
The picture can be found on the page Triply connected graph embeddings and the forest was constructed in a paper by S.T. Hyde and C. Oguey.