The mathematical beauty of snowflakes

Winter is coming. And with it, snowflakes will probably soon start to fall. These cold droplets have an incredibly beautiful, complicated and symmetrical structure. What creates these seemingly unique structures? And why are they symmetrical? What is symmetry? On the occasion of this time of the year, Ana Ros Camacho, mathematician at Utrecht University, sheds some light on the appearance of these very special, delicate little things.

Turning snowflakes

“If you were to grab the usual 6-branch snowflake, put it under a microscope and turn it 60 degrees, it would look as if nothing changed,” Ros Camacho starts. “This is called symmetry. In other words, symmetry is the property that something does not change under some transformation, like a rotation of 60 degrees. There are many other objects with a similar kind of symmetry as snowflakes, which, more specifically, is called rotational symmetry. A clock without numbers for instance, or the pattern on some of the Danish krone coins (image). The first time I visited Denmark I noticed the symmetry immediately!”

There are many other objects with a similar kind of symmetry as snowflakes.

Symmetry is everywhere

“Symmetry is all around us, and when you pay attention, you see it everywhere”, Ros Camacho adds enthusiastically. “You may find it in nature, in the shape of leaves, fruits and plants. Or in architecture, music and literature. In science, symmetry helps scientists understand so many things, such as some properties of molecules. In mathematics, more specifically, symmetry occurs in almost every branch: from geometry to algebra. It's even found in our common perception of being attractive, which is usually related to how symmetric the face of a person is. That, by the way, is not an instance of rotational symmetry, but of reflectional symmetry: the left side of a face is a reflection of the right side, and the other way around.”

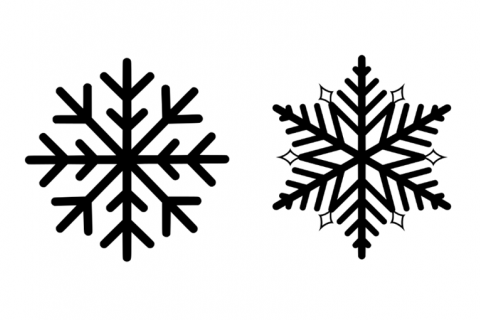
Different but similar

“They say that no two snowflakes are alike,” Ros Camacho continues. “But mathematics does not provide an answer to why their structures are so complicated. The shape of a snowflake is the result of many factors, such as the temperature and humidity at which it is formed. From a mathematical point of view, a lot of snowflakes are actually pretty similar: although the degree may vary (maybe you have to turn it 1/4 (left) of a turn instead of 1/6 (right)), nearly all snowflakes are rotationally symmetric.”

Going home for Christmas

“Rotational and reflectional symmetries can be used to create geometric patterns, including a third type of symmetry, which is called ‘translational symmetry’,” Ros Camacho says. “We often see it in wallpaper or tiles, for instance. And although the number of geometric patterns seems endless, mathematicians have proven the amazing fact that there are only seventeen unique patterns. Which means that every two-dimensional geometric pattern is derived from these seventeen patterns. The Spanish city of Granada is home to a very rare location where all seventeen patterns can be detected. It is the Alhambra, which is very special to me as an Andalusian mathematician. I try to visit it every year when I go back home for Christmas.”